Tetrahedron
Definition and Basic Properties
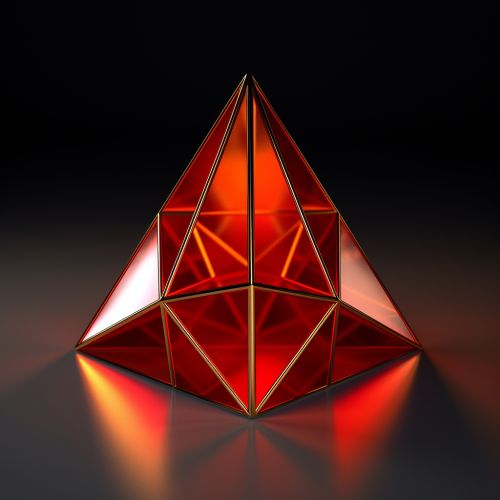
A tetrahedron is a polyhedron composed of four triangular faces, six straight edges, and four vertex corners. It is the simplest of all the ordinary convex polyhedra and the only one that has fewer than 5 faces. The tetrahedron is the three-dimensional case of the more general concept of a Euclidean simplex.

Geometry
The tetrahedron is one kind of pyramid, which is a polyhedron with a flat polygon base and triangular faces connecting the base to a common point. In the case of a tetrahedron, the base is a triangle (any of the four faces can be considered the base), so a tetrahedron is also known as a "triangular pyramid".
The tetrahedron is the only convex polyhedron that has four faces. The faces of a tetrahedron are equilateral triangles; that is, all four faces are congruent (identical in shape and size). The tetrahedron is one kind of convex polyhedron.
Tetrahedron in Nature and Culture
Tetrahedrons occur in many configurations, from natural crystal structures to man-made spacecraft. The regular tetrahedron also appears in the carbon atom, which forms covalent bonds in the shape of a tetrahedron. Tetrahedrons are also found in the structure of certain proteins.
In ancient cultures, the tetrahedron was considered the most sacred of all the shapes. The Pythagoreans revered the tetrahedron, as they believed it represented the element of fire. In modern times, the tetrahedron remains a symbol of change and transformation.
Mathematical Properties
The tetrahedron has many interesting mathematical properties. It is the simplest example of a topological space that can be given a differentiable structure, and it can be used to construct a variety of more complex spaces, including the four-dimensional Hypercube and the six-dimensional octahedron.
The volume of a tetrahedron is given by the formula V = 1/6abcSin(θ), where a, b, and c are the lengths of the edges, and θ is the angle between the vectors of any two edges. This formula is a special case of the more general formula for the volume of a pyramid.
