Linear equation
Definition
A linear equation is a mathematical statement that performs a relation between two variables using a linear function. The general form of a linear equation in two variables, x and y, is ax + by = c, where a, b, and c are constants, and a and b are not both zero. The equation represents a straight line when graphed in a two-dimensional plane.
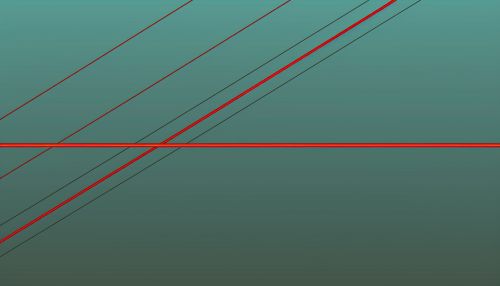
Properties
Linear equations have several distinct properties. They include:
- The graph of a linear equation is a straight line.
- The slope of the line is equal to the coefficient of x.
- The y-intercept of the line is the constant term of the equation.
- Two lines represented by linear equations are either parallel, intersecting, or coincident.
Solving Linear Equations
Solving a linear equation involves finding the set of values that make the equation true. For a linear equation in one variable, the solution is the value of the variable. For a linear equation in two variables, the solution is a set of ordered pairs (x, y) that satisfy the equation.
Systems of Linear Equations
A system of linear equations is a set of two or more linear equations with the same variables. The solution to a system of linear equations is the set of values that satisfy all the equations in the system simultaneously. There are several methods for solving systems of linear equations, including substitution, elimination, and matrix methods.
Linear Equations in Matrix Form
Linear equations can also be represented in matrix form. A system of linear equations can be written as a single matrix equation, which can then be solved using various matrix operations.
Applications
Linear equations have numerous applications in various fields, including physics, engineering, economics, and computer science. They are used to model and solve problems involving constant rates of change.
