Fano plane
Definition and Overview
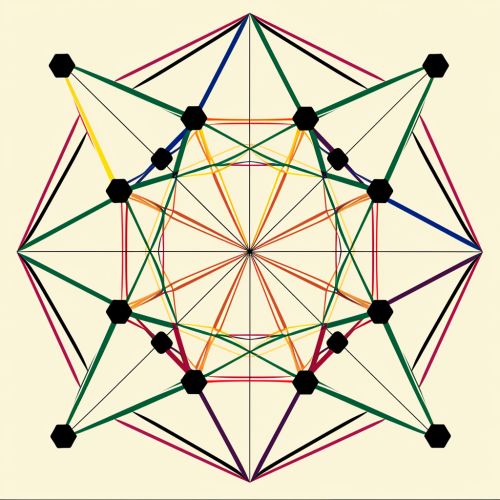
The Fano plane, also known as the Fano 7-point projective plane, is the smallest non-trivial projective plane. Named after Italian mathematician Gino Fano, it is a finite projective plane of order 2, meaning it contains seven points and seven lines, with three points on every line and three lines through every point.
Mathematical Description
The Fano plane can be defined mathematically as a set of seven points, along with a set of seven triples of points, called lines. Each line contains exactly three points, and each pair of distinct points belongs to exactly one line. This configuration can be represented using incidence matrices or incidence structures.
Properties
The Fano plane has several distinctive properties that set it apart from other projective planes. These include self-duality, meaning it is isomorphic to its dual plane, and the property of being a Steiner triple system, which is a set of triples with the property that each pair of elements appears in exactly one triple.
Applications
The Fano plane has applications in various fields of mathematics, including combinatorics, finite geometry, and algebra. It is used as a basic example in projective geometry due to its simplicity and symmetry. It also has applications in the design of block designs, which are combinatorial designs for experiments.
History
The Fano plane is named after Gino Fano, who was a leading figure in the development of finite geometry. However, the concept of the Fano plane predates Fano and can be traced back to the work of earlier mathematicians.
See Also